equinox; you then have the star located on the time
diagram. Let’s say it is the star Vega, whose SHA is
approximately 81°. Figure 15-9 shows Vega located on
the time diagram.
Itis easy to see herethat the GHA of Vega must be
equal to the GHA of the vernal equinox plus the SHA of
Vega (or GHAEL%%, = GHAr + SHAVqa. In this example,
the GHA of Vega is 81° plus 45°, or 126°.
Now let’s use the time diagram to explain some
more facts about nautical astronomy.
Local Hour Angle (LHA)
Local hour angle (LHA) is the name given to the
angle of arc (expressed in degrees, minutes, and tenths
of minutes) of the celestial equator between the celestial
meridian of a point on the celestial sphere and the hour
circle of a heavenly body. It is always measured
westward from the local meridian through 360°.
Let’s work this problem of LHA on a time diagram.
Say you are at longitude 135° from M toward
Greenwich which means, of course, that Greenwich
will be shown east of M. Think it over for a
moment—you are to the west of Greenwich; therefore,
Greenwich is to the east of you.
Now that we know where Greenwich is and where
you are, let’s figure the LHA of the sun as it is shown in
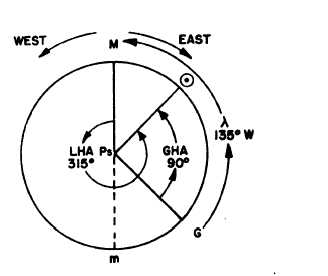
figure 15-8. Figure 15-10 shows us that the sun is 90°
west of Greenwich. We know that the LHA is always
measured westward from your location meridian(M) to
the hour circle of the body (in this example, the sun).
Therefore, the LHA here is the whole 360° around
minus the 45° between the sun’s hour circle and M. This
45° may be found by inspecting figure 15-10 or by
subtracting 90° from 135°. Let’s think this over—we are
135°W of Greenwich; therefore, G is 135° clockwise
from us. The sun is 90°W or counterclockwise from G.
The difference is the 45° we mentioned. Subtract this
45° from 360° and we get 315°, the LHA.
Look again at figure 15-10. As you can see, the sun
is east (clockwise on the diagram) of your local meridian
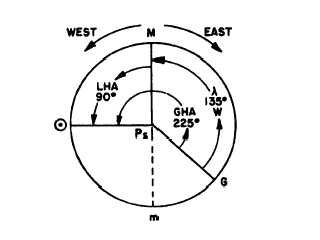
(M). Now let’s suppose that you are at the same
longitude (135°W), but the GHA of the sun is 225°
instead of 90°. The time diagram will appear as shown
in figure 15-11. ‘The sun is now west of your meridian
(M). The LHA is always measured westward from the
local celestial meridian to the hour circle of the body.
Therefore, the LHA is the 90° from M to the sun’s hour
circle.
Here are two general rules that will help you in
finding the LHA when the GHA and longitude are
known:
Figure 15-10.-LHA on the time diagram.
Figure 15-11.-LHA with the sun west of your celestial meridian.
1. LHA= GHA– AW (used when longitude is west)
2. LHA = GHA + kE (used when longitude is east)
In west longitude it may be necessary to add 360°
to the GHA before the subtraction can be made. In east
longitude, 360° is subtracted from the LHA if it exceeds
this amount. Be sure, however, to check the accuracy of
your work by referring to a time diagram. It offers a
graphic means of obtaining the data you need.
As an illustration, suppose the GHA of the sun is
and the longitude is 79°15’05”E. Since
longitude is east, you use formula 2 above. Transposing
to solve for the LHA, you have
15-13