line from transit setup A through piles 1, 2, 5, 10, 16,
and 25. AB measures 50/sin 60°40´, or 57.35 feet. This,
then, is the distance between adjacent transit setups on
the base line.
The distance from the base line to the first offshore
pile in any line also may be determined by
right-triangle solution. For pile No. 1 this distance is
prescribed as 50 feet. For piles 2, 3, and 4, first solve
the triangle A2L for 2L, which is 100/tan 29°20´, or
177.95 feet. The distance from 2 to Q is 150 feet;
therefore, QL measures 177.95 – 150, or 27.95 feet.
QD amounts to 27.95/tan 60°40´, or 15.71 feet.
Therefore, the distance from transit setup D to pile No.
8 is 50 + 15.71, or 65.71 feet. Knowing the length of
QL and the distance from setup point B to pile No. 3
by solving the right triangle LB3 for B3.
You can determine the distance E9 by solving the
determine the distance F15, G22, and H23 by solving
the right triangle AN10 and proceeding as before. For
pile No. 24, the distance I24 amounts to 50 tan 29°20´,
or 28.10 feet.
OFFSHORE LOCATION BY
TRIANGULATION
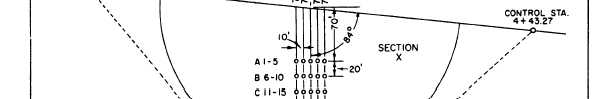
For piles located farther offshore, the
triangulation method of location is preferred. A pile
location diagram is shown in figure 10-30. It is
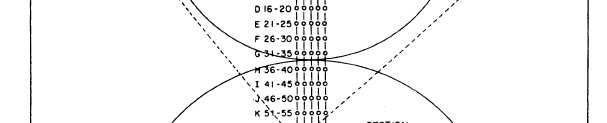
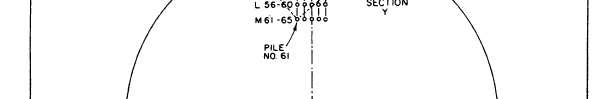
presumed that the piles in section X will be located by
the method just described, while those in section Y will
be located by triangulation from the two control
stations shown.
The base line measures (1,038.83 – 433.27), or
595.56 feet, from control station to control station.
The middle line of piles runs from station 7 + 41.05,
right triangle M5A and proceeding as before. You can
making an angle of 84° with the base line. The piles
Figure 10-30.—File location diagram.
10-28