STADIA INTERVAL.— As you look at a stadia rod
through a transit telescope, the stadia hairs seem to
intercept an interval on the rod. The distance on the rod
between the apparent positions of the two stadia hairs is
the stadia interval or stadia reading.
Usually, you determine stadia intervals by sighting
the lower stadia hair at a convenient foot mark and
then observing the position of the upper stadia hair;
for example, the lower hair might be sighted on the
2.00 foot mark and the upper hair might be in line
with 6.37. By subtracting, we have the stadia reading
(6.37 -2.00 = 4.37).
It may happen that the stadia reading is more than
the length of the rod. By using the middle hair, you may
observe a half-interval and multiply it by 2 to get the
stadia reading.
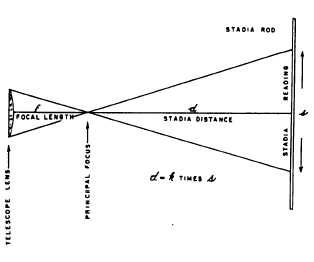
STADIA CONSTANT.— Light rays that pass
through the lens (objective) of a telescope come together
at a point called the principal focus of the lens. Then
these light rays continue in straight-line paths, as shown
in figure 8-3.
The distance between the principal focus and the
center of the lens is called the focal length(f) of the lens.
For any particular lens, the focal length does not change.
If you divide the focal length by the distance between
the stadia hairs (i), you get a number known as the stadia
constant (k). Sometimes the stadia constant is called the
stadia factor or stadia interval factor.
A convenient value to use for the stadia constant is
100. Stadia hairs usually are spaced so that the interval
between them will make the stadia constant equal to
100.
STADIA DISTANCE.— The distance from the
principal focus to the stadia rod is called the stadia
distance. As shown in figure 8-3, this distance (d) is
Figure 8-3.—Light rays converge at principal focus of a lens.
equal to the stadia constant (k) times the stadia reading
(s).
INSTRUMENT CONSTANT.— The distance
from the center of the instrument to the principal focus
is the instrument constant. Usually, this constant is
determined by the manufacturer of the instrument. You
should find it stated on the inside of the instrument box.
Externally focusing telescopes are manufactured so
that the instrument constant may be considered equal to
1. For internally focusing telescopes, though, the
objective in the telescope is so near the center of the
instrument that the instrument constant may be
considered as zero. This, as you will learn in the
following discussion of stadia reduction formulas, is a
distinct advantage of internally focusing telescopes.
Most modem instruments are equipped with internally
focusing telescopes.
STADIA REDUCTION FORMULAS.— In stadia
work we are concerned with finding two values as
follows: (1) the horizontal distance from the center of
the instrument to the stadia rod and (2) the vertical
distance, or difference in elevation, between the center
of the instrument and middle-hair reading on the rod. To
obtain these values, you must use stadia reduction
formulas.
Stadia Formula for Horizontal Sights.— For a
horizontal sight, the distance that we need to determine
is the horizontal distance between the center of the
instrument and the stadia rod. This distance is found by
adding the stadia distance to the instrument constant as
follows:
Write ks for the stadia distance and (f + c) for the
instrument constant. Then the formula for computing
horizontal distances when the sights are horizontal
becomes the following:
Where:
h
=
k
=
s
=
f + c =
f =
c
=
horizontal distance from the center of the
instrument to a vertical stadia rod
stadia constant, usually 100
stadia interval
instrument constant (zero for internally
focusing telescopes; approximately 1 foot
for externally focusing telescopes)
focal lengths of the lens
distance from the center of the instrument to
the center of the lens
8-4