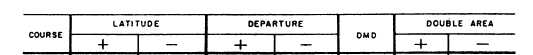
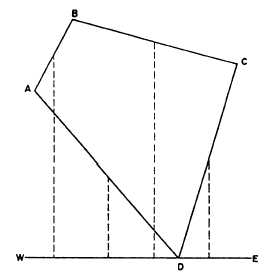
Figure7-21.—Area from double meridian distances.
For convenience, it is customary to use double
meridian distance (DMD) rather than meridian
distance in calculations. When the meridian distance of
the initial traverse line in a closed traverse equals one
half of the departure of the line, the DMD of this line
equals its departure. Again, from the rule for meridian
distance of the next line, the DMD of that line equals the
DMD of the preceding line, plus the departure of the
preceding line, plus the departure of the line itself.
It can be shown geometrically that the area
contained within a straight-sided closed traverse equals
the sum of the areas obtained by multiplying the
meridian distance of each traverse line by the latitude of
that line. Again the result is the algebraic sum. If you
multiply a positive meridian distance (when the
reference meridian runs through the most westerly
station, all meridian distances are positive) by a plus or
north latitude, you get a plus result that you add. If you
multiply a positive meridian distance by a minus or
south latitude, however, you get a minus result that you
subtract.
Therefore, if you multiply for each traverse line the
double meridian distance by latitude instead of meridian
distance by latitude, the sum of the results will equal
twice the area, or the double area. To get the area, you
simply divide the double area by 2.
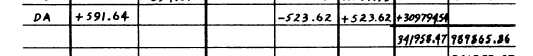
Figure 7-21 shows entries for the computations of
the DMD of the area of the traverse we have been
working on. Because AB is the initial traverse line, the
DMD of AB equals the departure. The DMD of BC
equals the DMD of AB (125.66), plus the departure of
AB (125.66), plus the departure of BC (590.65), or
841.97 feet. The DMD of CD equals the DMD of BC
(841.97), plus the departure of BC (590.65), plus the
departure of CD (which is minus 192.69, and therefore
is subtracted), or 1239.93 feet. The DMD of DA equals
the DMD of CD (1239.93), plus the departure of CD
(–192.69), plus the departure of DA (–523.62), or 523.62
feet. Note that the DMD of this last traverse line equals
the departure of the line, but with an opposite sign. This
fact serves as a check on the computations.
The double area for AB equals the DMD times the
latitude or
125.66 x 255.96 = 32,163 .93square feet.
The double area for BC equals 841.97 (the DMD) times
minus 153.53 (the latitude), or minus 129,267.65
square feet. The double area of CD is
1,239.93 x (-694.07) = –860,598.21 square feet.
Figure 7-22.—Parallel distances.
7-16