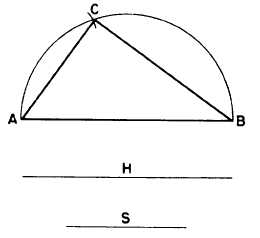
Figure 4-13.-Constructing a right triangle with hypotenuse
and one side given.
EQUILATERAL TRIANGLE:
LENGTH OF SIDE GIVEN
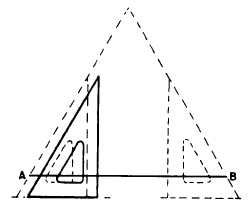
To construct an equilateral triangle when the
length of a side is given, you can follow the
method previously described for constructing a
triangle when the length of each side is given. The
sides of an equilateral triangle are equal in length.
Each angle in an equilateral triangle measures
60°. This fact is applied in the method of
constructing an equilateral triangle with given
length of side, such as the one shown in
figure 4-14. Simply use a 30°/60° triangle
and a T square or straightedge to erect lines from
A and B at 60° to AB.
EQUILATERAL TRIANGLE IN A
GIVEN CIRCUMSCRIBED CIRCLE
A circumscribed plane figure is one that
encloses another figure, the circumscribed figure
being tangent to the extremities of the enclosed
figure. An inscribed plane figure is one that is
enclosed by a circumscribed figure.
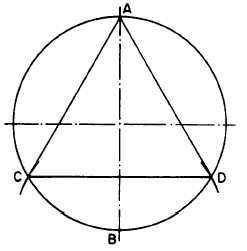
Figure 4-15 shows you how to inscribe an
equilateral triangle within a given circumscribed
circle. Draw a vertical center line intersecting the
given circle at A and B. With B as a center and
a radius equal to the radius of the circle, strike
arcs intersecting the circle at C and D. Lines
connecting A, C, and D form an equilateral
triangle.
EQUILATERAL TRIANGLE ON A
GIVEN INSCRIBED CIRCLE
Figure 4-16 shows one method of circum-
scribing an equilateral triangle on a given inscribed
Figure 4-14.-Equilateral triangle with a given length of
side AB.
Figure 4-15.-Equilateral triangle in a given circumscribed
circle.
Figure 4-16.-Equilateral triangle on a given inscribed
circle: one method.
4-5